Solutions and concentrations
In industrial chemistry, the concentrations of chemical solutions used may be critical to the success of the process. If the concentration is high, the reaction may be too fast, or other unwanted reactions will occur. A low concentration may result in a rate of reaction that is too slow to make the process viable. The concentration of the product may also have to match the customer's criteria, so dilution of the initial product may be required.
Concentration calculations
Remember that the concentration of a solution in moles per litre (mol L-1) can be given by the relationship shown below.
c=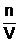
which can be rearranged to give: n = cV
Dilution of solutions
If the concentration of a solution needs to be changed, it is normally done by adding more of the solvent to dilute the solution. It is possible to calculate the new concentration by using the relationship shown below.
c1V1 = c2V2
c1 and c2 are the concentrations before and after any change, and V1 is the initial volume with V2 being the final total volume of the solution.
This relationship works because, as you have seen above, the number of moles of the solute is given by the relationship n = cV. When the total volume of the solution changes, the amount of solute remains the same, so whatever changes are made, concentration © multiplied by volume (V) will give a constant value, which leads to the relationship shown above.
This relationship can be rearranged to give the following.
c2 =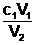
or V2 =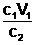 
Note that in using these relationships, any units of concentration and volume can be used, provided they are consistent within the problem.
Click here to see an example
What is the final concentration when 480 mL of water is added to 120 mL of hydrochloric acid with a concentration of 2.50 mol L-1?
Hint: Always write down the known values.
c1 = 2.50 mol L-1
V1 = 120 mL
c2 = unknown
V2 = 120 mL + 480 mL = 600 mL
c2 =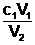
= 
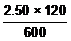= 0.500 mol L-1Now watch a video example of this type of problem. The question answered in the video is:
How much 8.0 mol L-1 sulfuric acid will be required to make 30 L of 1.5 mol L-1 sulfuric acid?
How much 8.0 moles per litre sulfuric acid will be required to make 30 litres of 1.5 moles per litre sulfuric acid?
The question asks how much of the sulfuric acid will be needed, so we can call this V1. Therefore, using the expression c1V1 = c2V2 , we can work out that V1 equals c2 multiplied by V2 and then divided by c1.
In this case this means that the volume required equals 1.5 multiplied by 30 divided by 8.0 which equals 5.6 litres.
Click here to open the video in a new window.
Give the new concentrations (in mol L-1) of the following solutions, type in your answers to three significant figures. Check your answer to each problem before moving on to the next question. Make sure you are careful with the volumes and read the feedback for each question.
1 |
2 |
3 |
4 |
Complete the following Solution concentration questions 
to practise these types of problems. Answers are given at the end so you can check your working.
